Coastal Vectors

Brisco County Jr. Orb Rod

In 1993, The Adventures of Brisco County Jr. premiered on Fox. It was about a Bounty Hunter in the old west, and his mission to round up the gang of outlaws who killed his father, a famous Marshal. Brisco County is a Harvard educated lawyer who is eagerly looking to the future for the “coming thing”.

As he works to capture all the outlaws responsible for his father’s death, he has multiple encounters with a mysterious object only known as ‘the orb’. In the first episode we learn that the orb can grant power to people who possess it. As we continue through the season, we learn more about the orb’s power and origins, but its mystery isn’t completely unraveled until nearly the end of the season.

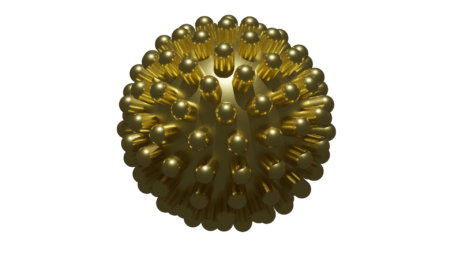
The orb is hard to describe. It’s a golden, spherical object with protrusions regularly distributed across it’s surface. These protrusions are the end-caps or glowing rods that can be removed from the orb. As a kid, I always thought that the orb’s mystery and power was very fantastic, and I always wanted to see it in person, or to own it.

Reference photo found on a prop auction site

I finally decided that it was time to replicate it. Not the whole thing, just one of the orb rods. I’ve done a bit of research here and there over the years and have never gotten a straight answer on the exact size of the prop. I managed to find a small image of a prop replica that was built from the same mold as the original prop. Luckily, this prop happened to be photographed next to a ruler. I inferred the measurements to the best of my ability and concluded that the prop orb rod is about 1.5 inches in diameter, and about 13.25 inches long.

Brisco County Orb Rod Plans

I was able to find blue acrylic rod in 1.5 inch diameter from a supplier on eBay for a good price. I then bought some Brass stock on Amazon, also 1.5 inches in diameter.

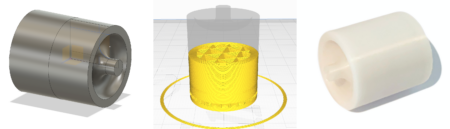
I don’t have any tooling for doing a rounded end of this diameter, so I used the lathe to create steps at 1/8 inch intervals in the brass that match the contour of a .75 inch radius sphere. I then used a file to smooth it down to a nice rounded end. A little bit of sanding, and then a clear coat of lacquer to prevent tarnishing, and the cap is finished. The reverse side was drilled out to 1 inch to accommodate the acrylic rod.

There wasn’t much to do on the acrylic rod, except to machine down one end in order to insert it into the cap. Additionally, the other end was fairly rough from being cut off with a band-saw by the seller. I used progressively finer sand paper on the end, finishing with a 2000 grit wet sanding to get it to be a clear and smooth as possible.

The last step was to epoxy the cap onto the acrylic rod.

Finally, after many decades, I have the prop replica I’ve always dreamed about.

Sadly, Brisco County Jr. was cancelled in its first season. Despite being cancelled, I find it to be an enduring show full of the witty one-liners you’d expect from Bruce Campbell, the charm of old spaghetti westerns, and a touch of sci-fi. Luckily, the show was able to satisfyingly conclude it’s storyline, so even while it was cancelled, it feels complete, and is one show that I frequently rewatch.

3D Printing

Way back when I was an itty bitty boy, I remember reading about rapid prototyping, and seeing pictures of objects created from an SLA printer. The object I remember most was a rook with an internal spiral staircase. Was was amazing about it is that it is an object that would be impossible to injection mold, or carve using a CNC machine. It had amazing detail for such a diminutive object.

When I went to college, we had a pair of powder bed printers that we could use in our manufacturing laboratory. Powder bed printing is extremely versatile because the objects being printed do not require any support structures. This allows for flexibility in design that could be difficult to achieve otherwise.

Since graduating, there has been an at-home 3D printing boom. I recall when the MakerBot went mainstream. It was very interesting, but I was very put off by the quality of the prints. The filament left a very jagged surface, there wasn’t a ton of detail, and the few prints I had seen in person had de-lamination issues in some of the layers. I’ve been very skeptical of filament printers since.

I finally gave in after seeing some prints a friend at work made using his printer. The prints still have the trademark layer lines of a filament printer, but the detail has improved so much. I got a Creality Ender 3 ($260 at time of writing) which seems so incredibly cheap. I am extremely impressed with what this machine can do.

Setup

The printer comes unassembled. It took about 30 minutes to put it together. It really required very little skill, as long as you know how to turn an Allen wrench.

Once it is assembled, the next thing to do is level the bed. This is accomplished with 4 knobs underneath the print bed. Again, it’s a pretty basic process.

Lastly, feeding the filament is a simple task. After that, it’s ready to print.

The first print I did was preloaded on the thumb drive. It was a little dog figurine. It printed perfectly!

Software

The printer came with it’s own branded software. Unfortunately, it is Windows only, but off the bat I was impressed by the ease of use. I’ve never seen or used slicing software before, but I was delighted by how intuitive and easy it is to use. It has basic presets as well as expert settings. For most things, the basic presets are great. I’ve used them for multiple projects without any real issue.

Replacement spool holder for a tape dispenser; Modeled in Fusion 360, Set up in slicer, printed in white PLA

I did eventually find out about Utilimaker’s Cura and downloaded it so I could slice on the Mac, and also so I could test tree supports. Cura had a built in printer profile for the Ender 3, which was great. My first few prints using it for slicing have also been a success.

Printing

Now, it’s not all great. I’ve had my share of failed prints. Many times this is do to positioning something sub-optimally, or trying to print something that’s just too small and delicate. In most cases I’ve been able to work around it by rotating or splitting the object up. Another issue I’ve had is with smaller items sticking to the print bed. Adding a raft certainly remedies these issues, however it uses a lot of extra material.

I did buy an upgraded glass print bed. The plastic mat that came with the printer already had visible signs of wear after just a month or two. The glass bed has been performing pretty well, The adhesion is good, and it seems to be resistant to wearing. I still have problems with larger prints pulling up around the base edges though.

Conclusion

I’ve always steered away from FDM printers because of their inherent drawbacks, such as requiring supports and the inherent layered texture of the printed piece. Despite all those misgivings, I am quite pleased with this product, and equally with it’s affordable price. One thing to be weary of though, I’ve found that with this hammer in my tool chest, most of my problems have started to look like nails.

Project Euler 117

I enjoy solving Project Euler problems in my downtime. Problem 117 is one that I’ve looked at multiple times, but never came up with a good solution. The premise is easy enough, but, as with most Euler problems, the scale of the problem presents issues.

Using a combination of grey square tiles and oblong tiles chosen from: red tiles (measuring two units), green tiles (measuring three units), and blue tiles (measuring four units), it is possible to tile a row measuring five units in length in exactly fifteen different ways.

How many ways can a row measuring fifty units in length be tiled?

For the context of my solution, I am representing the tiles as an array or list of integers that each represent the length of a tile. For example, (1, 3, 1) would be a black, green, and black tile taking up a total of 5 spaces.

Brute Force sometimes works on these problems, but more often than not, they take far too long to complete. I don’t feel like I’ve “solved” one of these problems if the solution takes more than a second or two. My first naive attempt to solve this problem was to attack it recursively and build all the combinations of tiles. After letting it run for a few minutes, I gave up and assumed there must be a better way.

While building each tile combination was prohibitively time consuming, I found that I could still use recursion to get the base combinations for the tiles. There are only about 1000 of those, so the trick will be permuting them to get the full count. Well, as it turns out, this led to a few more problems.

Using the permutations tool provided in itertools proved to be too slow as well. First of all, it was finding too many solutions. For example:
>>> for i in list(itertools.permutations([1, 1, 2, 2])): print i ... (1, 1, 2, 2) (1, 1, 2, 2) (1, 2, 1, 2) (1, 2, 2, 1) (1, 2, 1, 2) (1, 2, 2, 1) (1, 1, 2, 2) (1, 1, 2, 2) (1, 2, 1, 2) (1, 2, 2, 1) (1, 2, 1, 2) (1, 2, 2, 1) (2, 1, 1, 2) (2, 1, 2, 1) (2, 1, 1, 2) (2, 1, 2, 1) (2, 2, 1, 1) (2, 2, 1, 1) (2, 1, 1, 2) (2, 1, 2, 1) (2, 1, 1, 2) (2, 1, 2, 1) (2, 2, 1, 1) (2, 2, 1, 1)
When in reality many of those are equivalent in the terms of the problem conditions. What we want is a set which looks more like this:
>>> for i in set(itertools.permutations([1, 1, 2, 2])): print i ... (1, 1, 2, 2) (2, 1, 2, 1) (2, 1, 1, 2) (1, 2, 2, 1) (1, 2, 1, 2) (2, 2, 1, 1)
That works, but the program still has to calculate all the permutations that we are throwing out which is time consuming; at the most, a set will have 50 tiles which is 50! permutations. That’s 30414093201713378043612608166064768844377641568960512000000000000 permutations, which is really just an insane amount! So that won’t do.

Well, we don’t need to know the permutations, just the amount of permutations! Let’s see if we can find a formula for that. The general formula that I found which researching permutations looks like this:

where n is the number of terms, and r is the number of terms you are choosing from your source pool. In this case, we are going to always use all the tiles in our base combination that we want to permute, so the number of permutations is just the factorial of the number of tiles. Hmm. That doesn’t really help since it doesn’t filter out any duplicates.

Perhaps if I researched a bit more, I could have found the proper formula for this situation. Rather than read more about permutations, I decided to take one of my base combinations and do some experiments to see if I could derive a formula for this situation. I took the last combination from my list and computed the number of unique permutations in order to see what my goal should be. (This operation took over 10 minutes)
>>> print len(set(itertools.permutations([3, 3, 4, 4, 4, 4, 4, 4, 4, 4, 4, 4, 4]))) 78
I played around with factorials and found that for my 13 terms there would be 13!, or 6227020800, possible permutations. Since I knew I wanted the formula to result in 78, I divided the number of permutations by 78 and got 79833600. In theory, this number would be the denominator of my not-yet-existent formula. I tried grouping my 3s and 4s together; there are 2 and 11 respectively. I checked what 11! is and it turned out to be 39916800 which is exactly half of my target value for the denominator. And 2! (for the number of 3s) is 2. That seems pretty promising!

My hypothesis was that multiplying the factorials of the number of similar terms together and then dividing them into the number of possible permutations would yield my desired result. I tested it out on another combination and it worked out correctly, so I modified my program to perform this operation on each combination. I ran it and checked the answer, and it was correct!

And it only took .083 seconds! I’m pretty happy with the results. I’m sure a mathematician might have a more clever, direct way of solving this problem, however I think that since this solution is accurate and quick I can be proud of it. You can see the complete code on my github.